Self-Potential (SP)

Basic Concept

The self-potential (SP) method is a passive electrical survey technique that, since the early twentieth century, was predominantly used for metallic sulfide ore prospecting. Because of advancements in the electrical resistivity and induced polarization methods, SP is not as frequently used as it was historically. However, it does provide significant and unique information that can be applied to modern environmental and hydrogeological investigations.

Self-potential is a naturally occurring potential difference (i.e., voltage) generated by subsurface current flow that arises in the absence of an external electric field (Beck, 1981). In some literature, this phenomenon may also be referred to as spontaneous potential, secondary potential, or spontaneous polarization. Measuring the strength and determining the distribution of these SP signals can help determine the causative sources and their contributing mechanisms.

Theory

The self-potential voltages measured at the earth surface are representative of underground charging mechanisms and can be produced in both natural and anthropogenically impacted media. Though measurable signals may result from unknown or a combination of factors, the voltage anomalies detected by the SP method can be used to infer subsurface processes.

The relevant SP-generating mechanisms are the electrokinetic and electrochemical phenomena produced under the influence of natural gradients in systems containing interfaces of electrically and/or chemically heterogeneous conductors. Such processes are typically related to the interactions between electrolytes (i.e., groundwater or pore fluids) and minerals.

Electrokinetic (or “streaming”) potential is generated in partially or fully saturated subsurface systems and influenced by groundwater flow through porous media. When in contact with groundwater, the mineral constituents of pore membranes often change chemically. Reactive surface sites of many common near-surface minerals undergo proton exchange with and/or sorption of dissolved ions from the porewater. These interactions form a fixed layer of charge (i.e., the compact layer) adjacent to the mineral surface.

The compact layer generates microscopic electric fields that electrically alter the nearby porewater by attracting oppositely charged ions (i.e., counterions) and repelling similarly charged ions (i.e., coions). This behavior creates a diffuse layer that coats the mineral-water interface. The diffuse layer is characterized by a surplus of counterions and deficit of coions relative to the free water nearer to the pore center (Revil and Jardani, 2013).

As groundwater is driven through the subsurface system, the diffuse layer becomes electrokinetically coupled to adjacent surface charges and resists the flow to which free water is subjected. Subsequently, the drag of the diffuse layer counterions polarizes the porewater and generates self-potential source currents. Such systems contain multiple dipoles, each of which has an orientation representative of local porewater flow.

Though the direction of polarity depends on mineral and water chemistry, individual dipoles are typically positive in the flow direction, especially when the electrolyte has a near neutral pH. Self-potential signals result from the flow-dependent distribution of microscale dipoles. The strength of the measured signal depends on the degree of electrokinetic coupling and distance from the electrodes to the points of current generation.

Electrochemical potential, which is the second major SP-generating mechanism can be responsible for higher magnitude SP anomalies. Such anomalies are often generated by a type of electrochemical potential that is historically associated with metallic ore prospection and referred to as the “mineralization” potential. These SP signals are typically related to reduction-oxidation (i.e., redox) reactions occurring in interconnected networks of ionically conductive groundwater and electronically conductive minerals.

Redox reactions involve the transfer of electrons from a reductant to an oxidant, whereby the reductant becomes oxidized and the oxidant becomes reduced. Redox potential is the measure of ease with which a material accepts electrons and generally decreases with depth as does the concentration of oxidants. Metallic minerals that connect regions of different redox potential serve as conduits through which electrons are transferred. This creates a circuit similar to a galvanic cell battery and an SP-signal generating current (Stoll and others, 1995).

Because current flows from high to low potential, these naturally occurring batteries are typically oriented sub-vertically. They often have an anode at depth and a near-surface cathode, the center of which produces the most negative SP measurement. This type of mineralization potential is commonly indicative of sulfide, oxide, or graphite ore bodies (Beck, 1981). However, there are other mineral-dependent SP sources that include the separation of charges that commonly occurs in clay-type minerals.

Applications

There are several strategies for conducting small and large scale as well as short and long term self-potential surveys, many of which are explained in depth by Revil and Jardani (2013). Often, a stationary electrode and roving electrode connected to a voltmeter via a robust, low resistance, and well-insulated wire are used to measure the voltage across numerous point pairs along the earth surface. Typically, the SP method employs nonpolarizing electrodes that allow current to freely pass through them without polarization.

Commonly, nonpolarizing porous pot electrodes are used in self-potential surveys. These are composed of porous-based cylinders in which a metal rod is immersed within a solution of one of its salts (e.g., copper rod suspended in copper sulfate solution). The porous material becomes saturated with the solution and allows for electrical connection between the metal rod and the ground (Mussett and Khan, 2000).

In the basic two-electrode setup, the stationary electrode is positioned at a base station, the location of which is chosen for its absence of present electrical noise. The roving electrode is moved intermittently between measurements, and the voltages between the two electrodes are measured and recorded. Because the method is susceptible to noise, the roving electrode should periodically reoccupy the base location to observe contact potential, dirty electrodes, drift, or other system changes (Wightman and others, 2003).

All SP measurements are relative to the base station. Thus, it is necessary to maintain measurement-point commonality through that specific base station. SP survey data can generate one-dimensional profiles or two-dimensional maps of potential difference distribution along survey transects or sites. Though, a typical SP survey delivers a contour map of the electrical potential distribution relative to the base station value.

Because the SP-producing mechanisms and their constraints are either not known or fully understood, quantitative analysis of the mechanism is typically avoided. Most self-potential investigations visually evaluate observed profile amplitudes or grid contour patterns and correlate them to known or estimated subsurface properties. However, certain qualitative estimates can be made.

The depth to an anomaly is roughly estimated by its half width, though the method is unlikely sensitive to zones exceeding 30 meters in depth. Therefore, SP is not a modern tool for ore prospection, as most of the shallow ores have already been discovered (Mussett and Khan, 2000). Alternatively, geometric source models can provide useful estimates of source configuration and gradients of pressure, temperature, or chemical concentration (Corwin, 1990).

The self-potential method has proved beneficial in the following applications:

Proxy flow sensor for monitoring subsurface gas or fluid movement and patterns
Mapping seepage flow associated with dams, dikes, reservoir floors, etc.
Mapping organic-rich contaminant plumes/delineate chemical concentration gradients
Geothermal exploration/subsurface thermal investigations
Qualitative ore body prospection
Concentration of ionic species in porewater
Archeologic investigations/ mapping anthropogenic alterations of native ground

Waterborne SP field data acquisition — Field set up of waterborne SP data collection. (Ikard et. al., 2018)

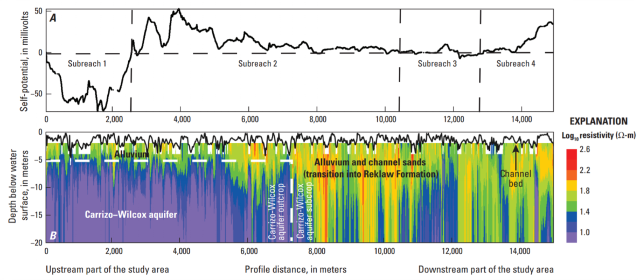
Example of SP results from waterborne survey. (Ikard et. al., 2018)

Examples/Case studies

Ahmed, A.S., Jardani, A., Revil, A., and Dupont, J.P., 2014, Hydraulic conductivity field characterization from the joint inversion of hydraulic heads and self‐potential data, Water Resources Research, v. 50, no. 4, p. 3502-3522, doi:10.1002/2013WR014645.

Abstract: Pumping tests can be used to estimate the hydraulic conductivity field from the inversion of hydraulic head data taken intrusively in a set of piezometers. Nevertheless, the inverse problem is strongly underdetermined. We propose to add more information by adding self‐potential data taken at the ground surface during pumping tests. These self‐potential data correspond to perturbations of the electrical field caused directly by the flow of the groundwater. The coupling is electrokinetic in nature that is due to the drag of the excess of electrical charges existing in the pore water. These self‐potential signals can be easily measured in field conditions with a set of the nonpolarizing electrodes installed at the ground surface. We used the adjoint‐state method for the estimation of the hydraulic conductivity field from measurements of both hydraulic heads and self potential during pumping tests. In addition, we use a recently developed petrophysical formulation of the streaming potential problem using an effective charge density of the pore water derived directly from the hydraulic conductivity. The geostatistical inverse framework is applied to five synthetic case studies with different number of wells and electrodes and thickness of the confining unit. To evaluate the benefits of incorporating the self‐potential data in the inverse problem, we compare the cases in which the data are combined or not. Incorporating the self‐potential information improves the estimate of hydraulic conductivity field in the case where the number of piezometers is limited. However, the uncertainty of the characterization of the hydraulic conductivity from the inversion of the self‐potential data is dependent on the quality of the distribution of the electrical conductivity used to solve the Poisson equation. Consequently, the approach discussed in this paper requires a precise estimate of the electrical conductivity distribution of the subsurface and requires therefore new strategies to be developed for the joint inversion of the hydraulic and electrical conductivity distributions.

Jardani, A., Revil, A., Bolève, A., Crespy, A., Dupont, J.P., Barrash, W., and Malama, B., 2007, Tomography of the Darcy velocity from self‐potential measurements, Geophysical Research Letters, v. 34, no. 24, 6 p., doi:10.1029/2007GL031907.

Abstract: An algorithm is developed to interpret self‐potential (SP) data in terms of distribution of Darcy velocity of the ground water. The model is based on the proportionality existing between the streaming current density and the Darcy velocity. Because the inverse problem of current density determination from SP data is underdetermined, we use Tikhonov regularization with a smoothness constraint based on the differential Laplacian operator and a prior model. The regularization parameter is determined by the L‐shape method. The distribution of the Darcy velocity depends on the localization and number of non‐polarizing electrodes and information relative to the distribution of the electrical resistivity of the ground. A priori hydraulic information can be introduced in the inverse problem. This approach is tested on two synthetic cases and on real SP data resulting from infiltration of water from a ditch.

Martínez-Pagán, P., Jardani, A., Revil, A., and Haas, A., 2010, Self-potential monitoring of a salt plume, Geophysics, v. 75, no. 4, p. 1JA-Z98, doi:10.1190/1.3475533.

Abstract: Nonintrusively monitoring the spread of contaminants in real time with a geophysical method is an important task in hydrogeophysics. We have developed a sandbox experiment showing that the self-potential method can locate both the source of leakage and the front of a contaminant plume. We monitored the leakage of a plume of salty water from a hole at the bottom of a small tank located at the top of a main sandbox. Initially, the sand was saturated by tap water. At a given time, a hole was opened at the bottom of the tank, allowing the salty water to migrate by diffusion and buoyancy-driven flow in the main sandbox. The bottom of the sandbox contained a network of 32 nonpolarizing silver-silver chloride electrodes with amplifiers, connected to a multichannel voltmeter. The self-potential response associated with the migration of the salt plume in the sandbox was recorded over time. A self-potential anomaly was observed with amplitude varying from a few millivolts at the start of the leak to a few tens of millivolts after a few minutes. The self-potential data were inverted using a time-lapse tomographic algorithm to reconstruct the position of the volumetric source current density over time. A positive volumetric source current density was associated with the position of the leak at the bottom of the leaking tank, whereas a negative volumetric source current density was associated with the salinity front moving down inside the sandbox. These poles were well reproduced by performing a finite-element simulation of the problem. Using this information, we estimated the speed of the salt plume sinking inside the sandbox. Therefore, the self-potential method can be used to track, in real time, the position of the front of a contaminant plume in a porous material.

Naudet, V., Revil, A., Bottero, J.Y., and Bégassat, P., 2003, Relationship between self‐potential (SP) signals and redox conditions in contaminated groundwater, Geophysical Research Letters, v. 30, no. 21, 4 p., doi:10.1029/2003GL018096.

Abstract: In situ measurements of redox potential are rather difficult to perform and provide only sparse information on its spatial distribution. To delineate redox fronts in a contaminant plume, the self‐potential (SP) method can be a helpful complement to geochemical measurements. Here, we apply the SP method to the Entressen municipal waste landfill (south‐eastern France) over a 20 km2 area. The results show a large negative SP‐anomaly of ∼−400 mV with respect to a reference station taken outside the contaminant plume. Once removed the electrokinetic component associated with groundwater flow, the residual self‐potential signals are linearly correlated with in situ measurements of redox potential. We propose a quantitative relationship between self‐potential and redox potential, which would be used to invert self‐potential measurements in terms of in situ redox potential values in contaminant plumes.

Revil, A., Karaoulis, M., Srivastava, S., and Byrdina, S., 2013, Thermoelectric self-potential and resistivity data localize the burning front of underground coal fires, Geophysics, v. 78, no. 5, p. 1SO-Z134, doi:10.1190/geo2013-0013.1.

Abstract: Self-potential signals and resistivity data can be jointly inverted or analyzed to track the position of the burning front of an underground coal-seam fire. We first investigate the magnitude of the thermoelectric coupling associated with the presence of a thermal anomaly (thermoelectric current associated with a thermal gradient). A sandbox experiment is developed and modeled to show that in presence of a heat source, a negative self-potential anomaly is expected at the ground surface. The expected sensitivity coefficient is typically on the order of −0.5 mV°C−1 in a silica sand saturated by demineralized water. Geophysical field measurements gathered at Marshall (near Boulder, CO) show clearly the position of the burning front in the electrical resistivity tomogram and in the self-potential data gathered at the ground surface with a negative self-potential anomaly of about −50 mV. To localize more accurately the position of the burning front, we developed a strategy based on two steps: (1) We first jointly invert resistivity and self-potential data using a cross-gradient approach, and (2) a joint interpretation of the resistivity and self-potential data is made using a normalized burning front index (NBI). The value of the NBI ranges from 0 to 1 with 1 indicating a high probability to find the burning front (strictly speaking, the NBI is, however, not a probably density). We validate first this strategy using synthetic data and then we apply it to the field data. A clear source is localized at the expected position of the burning front of the coal-seam fire. The NBI determined from the joint inversion is only slightly better than the value determined from independent inversion of the two geophysical data sets.

Panthulu, T.V., Krishnaiah, C., and Shirke, J.M., 2001, Detection of seepage paths in earth dams using self-potential and electrical resistivity methods, Engineering Geology, v. 59, no. 3-4, p. 281-295, doi:10.1016/S0013-7952(00)00082-X.

Abstract: Earth and rockfill dams are designed to operate under steady state seepage. Anomalous seepage may be a threat to the integrity of the structure. In spite of advances made in the fields of geotechnical engineering, it is not possible to have 100% leak-proof structure. Any excessive and unplanned seepage may lead to the failure of the dam, especially in unconsolidated or fractured terrains. Geophysical methods play an important role in mapping seepage paths and monitoring the changes of the seepage with time, enabling to plan technically and economically worthwhile remedial measures. In the present paper, utilisation of electrical methods for delineation of seepage zones at two of the four Saddle dams of the Som-Kamla-Amba project, Rajasthan State, India; which is founded on heterogeneous rock mass, is discussed. Electrical resistivity method was used to delineate zones favourable for seepage, whereas, self-potential (SP) method was used to delineate the seepage paths. SP measurements have shown negative anomaly of the order of 10–20 mV in amplitude, indicating low seepage, coinciding with the seepage measurements made by the project authorities.

Robert, T., Dassargues, A., Brouyère, S., Kaufman, O., Hallet, V., and Nguyen, F., 2011, Assessing the contribution of electrical resistivity tomography (ERT) and self-potential (SP) methods for a water well drilling program in fractured/karstified limestones, Journal of Applied Geophysics, v. 75, no. 1, p. 42-53, doi:10.1016/j.jappgeo.2011.06.008.

Abstract: ERT and SP investigations were conducted in carbonate rocks of the Dinant Synclinorium (Walloon Region of Belgium) to find suitable locations for new water wells in zones with little hydrogeological data. Since boreholes information needed to be representative of the area, large fractured zones were searched for the drillings. Large ERT profiles (320 to 640 m) allowed us to image the resistivity distribution of the first 60 m of the subsurface and to detect and characterize (in terms of direction, width and depth) fractured zones expected to be less resistive. Data errors, depth of investigation (DOI) indexes and sensitivity models were analyzed in order to avoid a misinterpretation of the resulting images. Self-potential measurements were performed along electrical profiles to complement our electrical results. Some negative anomalies possibly related to preferential flow pathways were detected. A drilling campaign was conducted according to geophysical results. ‘Ground truth’ geological data as well as pumping tests information gave us a way to assess the contribution of geophysics to a drilling program. We noticed that all the wells placed in low resistivity zones associated with SP anomalies provide very high yields and inversely, wells drilled in resistive zones or outside SP anomalies are limited in terms of capacity. An apparent coupling coefficient between SP signals and differences in hydraulic heads was also estimated in order to image the water table.

References

Beck, A.E., 1981, Self-potential or spontaneous polarization, in Physical Principles of Exploration Methods: London, Macmillan Publishers Limited, p. 31-41.

Corwin, R.F., 1990, The Self-Potential Method for Environmental and Engineering Applications, in Ward, S.H, ed., Geotechnical and Environmental Geophysics: Society of Exploration Geophysics, v. 1, p. 127-146.

Ikard, S.J., Banta, J.R., and Stanton, G.P., 2018, New insights into surface-water/groundwater exchanges in the Guadalupe River, Texas, from floating geophysical methods: U.S. Geological Survey Fact Sheet 2018–3057, 4 p., https://doi.org/10.3133/fs20183057.

Martínez-Pagán, P., Jardani, A., Revil, A., and Haas, A., 2010, Self-potential monitoring of a salt plume, Geophysics, v. 75, no. 4, p. 1JA-Z98, doi:10.1190/1.3475533.

Mussett, A.E. and Khan, M.A., 2000, Induced Polarisation and Self-Potential, in Looking Into the Earth: An Introduction to Geological Geophysics: New York, Cambridge University Press, p. 202-209.

Revil, A. and Jardani, A., 2013, The Self-Potential Method: Theory and Applications in Environmental Geosciences: New York, Cambridge University Press, 369 p.

Revil, A., Naudet, V., Nouzaret, J., and Pessel, M., 2003, Principles of electrography applied to self‐potential electrokinetic sources and hydrogeological applications, Water Resources Research, v. 39, no. 5, 15 p., doi:10.1029/2001WR000916.

Stoll, J., Bigalke, J., and Grabner, E.W., 1995, Electrochemical modelling of self-potential anomalies, Surveys in Geophysics, v. 16, no. 1, p. 107-120.

Wightman, W.E., Jalinoos, F., Sirles, P., and Hanna, K., 2003, Application of Geophysical Methods to Highway Related Problems: Lakewood, CO, Federal Highway Administration, Office of Bridge Technology, 742 p.

Wynn, J.C. and Sherwood, S.I., 1984, The Self-Potential (SP) Method: An Inexpensive Reconnaissance and Archaeological Mapping Tool, Journal of Field Archaeology, v. 11, no. 2, p. 195-204, doi:10.1179/jfa.1984.11.2.195.